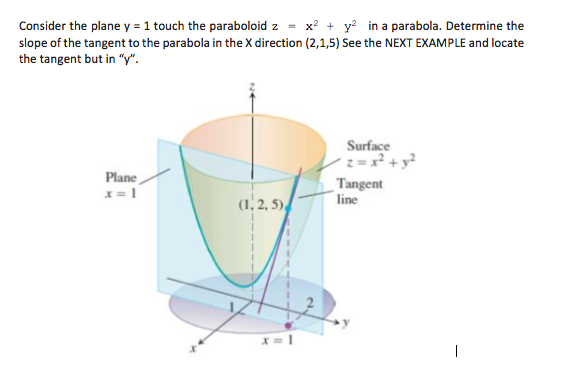
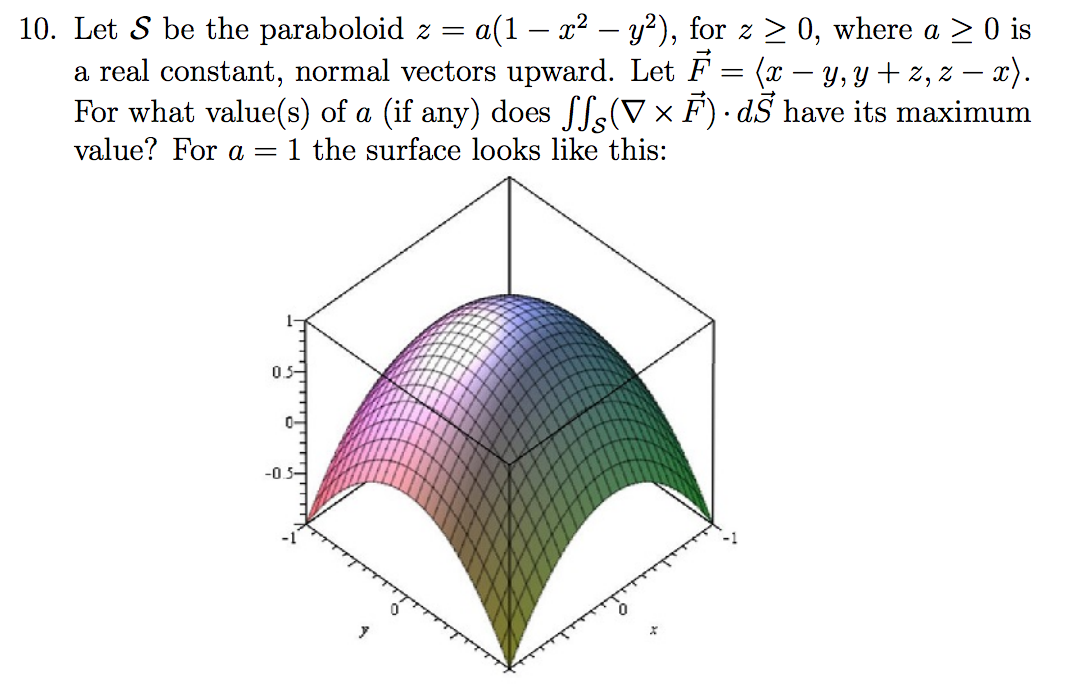
Here we shall aim at understanding some of the important properties and terms related to a parabola Tangent The tangent is a line touching the parabola The equation of a tangent to the parabola y 2 = 4ax at the point of contact \((x_1, y_1)\) is \(yy_1 = 2a(x x_1)\) Normal The line drawn perpendicular to tangent and passing through the point of contact and the focus of the
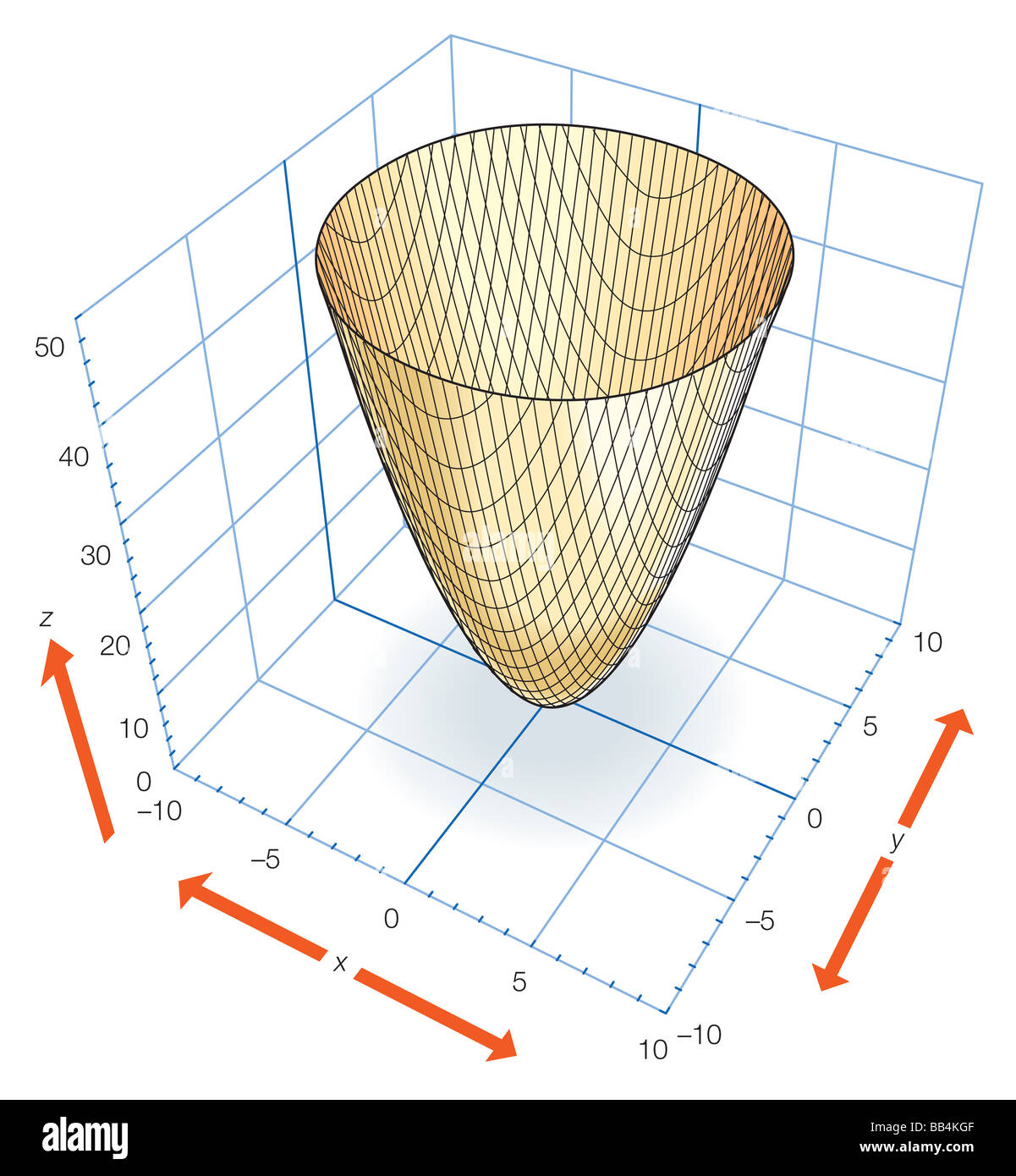
The part of the paraboloid z=1-x^2-y^2- Where does the normal line to the paraboloid z = x^2 y^2 at the point (1, 1, 2) intersect the paraboloid a second time?Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history
The part of the paraboloid z=1-x^2-y^2のギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 |  | |
 |  | |
 |  | |
 |  | |
「The part of the paraboloid z=1-x^2-y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 |  | |
 |  | |
「The part of the paraboloid z=1-x^2-y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 |  |  |
 | ||
 |  |  |
「The part of the paraboloid z=1-x^2-y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 |  | |
 | ||
 |  |  |
「The part of the paraboloid z=1-x^2-y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 | ||
 | ||
 |  | |
「The part of the paraboloid z=1-x^2-y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 |  | |
 |  | |
「The part of the paraboloid z=1-x^2-y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | |
 |  | |
 |  |  |
「The part of the paraboloid z=1-x^2-y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 | ||
 | ||
 | ||
「The part of the paraboloid z=1-x^2-y^2」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
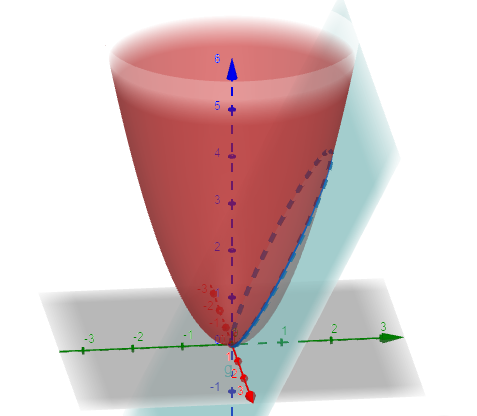 |  |
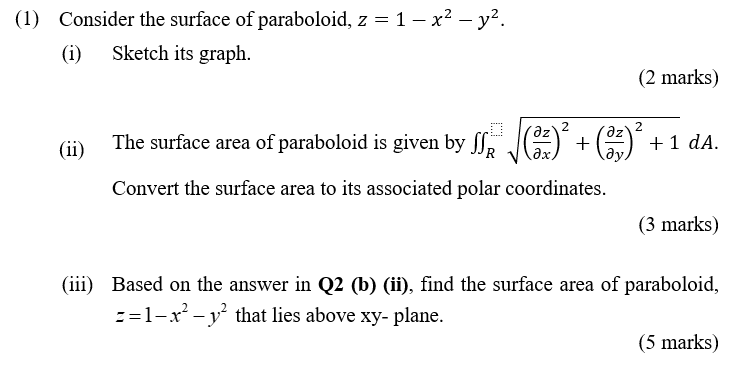
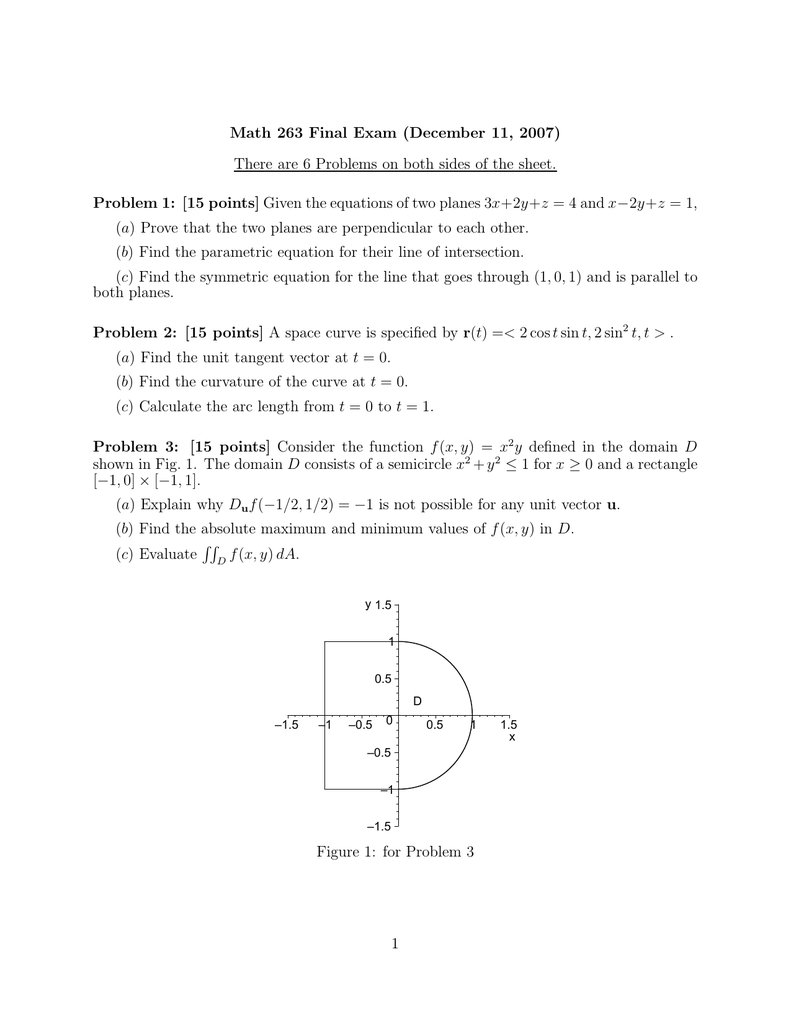
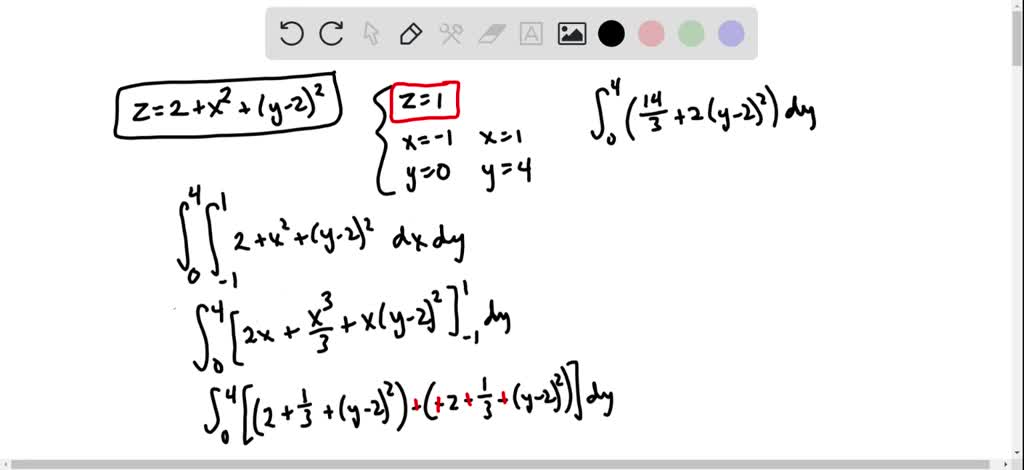
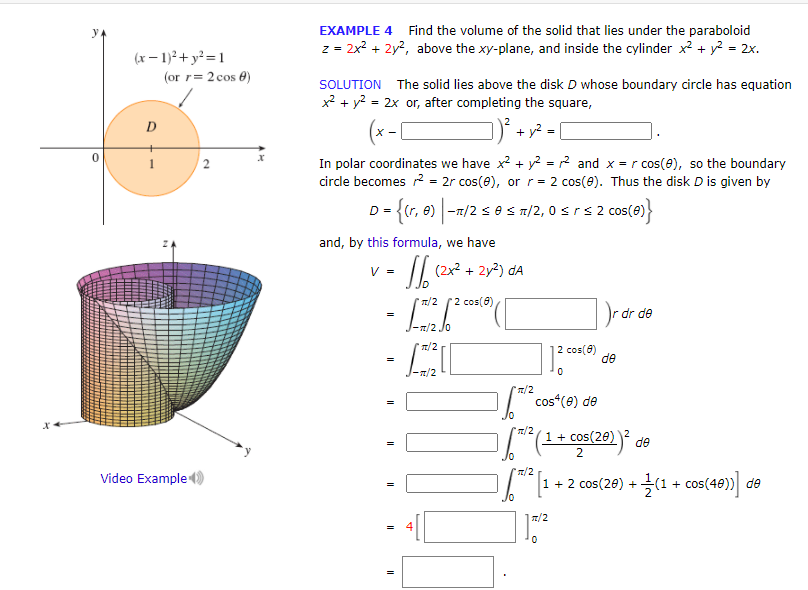
1 x2 y2dA= Z 2ˇ 0 Z 1 0 1 r2 = 2ˇ 3 (2 2 1) 4) Find the area of the nite part of the paraboloid y= x 2 z cut o by the plane y= 25 Solution Surface lies above the disk x 2 z in the xzplane A(S) = Z Z D p f2 x f z 2dA= Z Z p 4x2 4y2 1da Converting to polar coords get Z 2How do you find the surface area of the part of the circular paraboloid ##z=x^2y^2## that lies inside the cylinder ##x^2y^2=1##?
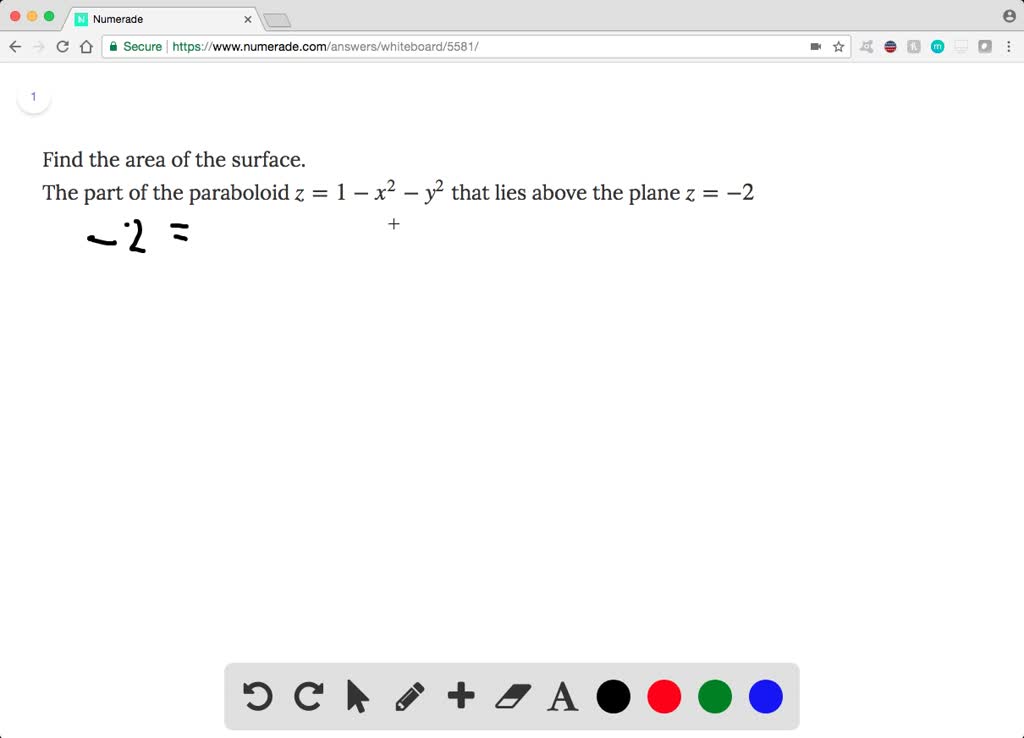
Incoming Term: paraboloid z=1-x^2-y^2, the part of the paraboloid z=1-x^2-y^2, enclosed by the paraboloid z=x^2+y^2+1, find the area of the surface. the part of the paraboloid z=1-x^2-y^2,



